Investment Return Calculation: The Ultimate Pro Guide

In today’s increasingly complex financial landscape, accurately calculating investment returns is the cornerstone of building sustainable wealth. Mastering investment return calculation gives investors the clarity to evaluate performance, compare opportunities, and make data-driven decisions that compound wealth over time.

Key Takeaways

Simple return calculations often mask the true performance of investments. When an investor earned 25% in year one and lost 20% in year two on a $10,000 investment, simple averaging suggests a 2.5% annual return, but the actual money-weighted return reveals a loss of 0.25% – a difference that compounds dramatically over decades.
Time-weighted return (TWRR) metrics eliminate the distortion caused by cash flows, making them essential for evaluating investment manager performance. A portfolio manager who achieved exceptional 15% annual returns might show disappointing results when measured with simple return methods if clients added significant capital before market downturns – TWR correctly shows their skill independent of timing decisions they didn’t control.
Different return metrics serve different purposes – using the wrong calculation for your specific need is a common but costly mistake. When comparing two investment opportunities, an angel investor correctly used IRR (Internal Rate of Return) to determine that a technology startup offering 40% IRR over five years was superior to a real estate project with 50% simple return over the same period, recognizing the impact of when returns were received.

Understanding Investment Returns: The Foundation of Wealth Building

Investment returns represent the profits or losses generated by an investment over a specific period, typically expressed as a percentage of the initial investment amount. While this definition seems straightforward, the calculation methods can vary significantly depending on the investment type, time horizon, and specific circumstances of the investor.

The accuracy of return calculations directly impacts wealth-building strategies by influencing asset allocation decisions, risk management approaches, and ultimately, the achievement of financial goals. Sophisticated investors recognize that different return metrics serve different purposes – simple return calculations might suffice for quick comparisons, while complex metrics like Internal Rate of Return (IRR) provide deeper insights for multi-year investments with irregular cash flows.

Understanding return calculations is not merely an academic exercise but a practical necessity for modern investors. According to a 2023 study by Vanguard, investors who accurately tracked and understood their portfolio returns outperformed those who didn’t by an average of 1.8% annually – a difference that compounds to over 60% greater wealth over 25 years.

power of compound returns in the theme investment return calculation

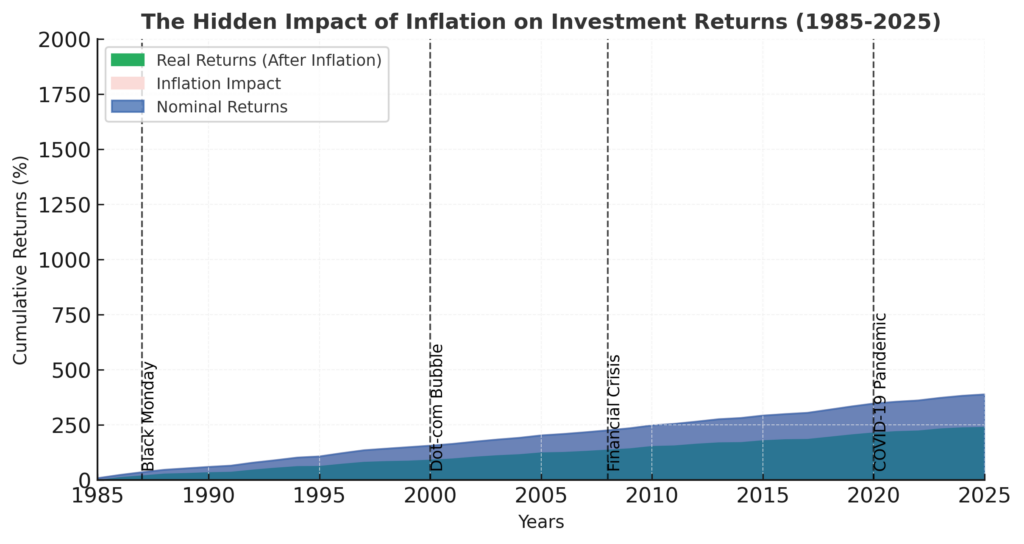
The distinction between nominal and real returns further complicates the picture. In 2023, an investment yielding a seemingly impressive 7% nominal return actually delivered only 2.5% in real purchasing power when accounting for the 4.5% inflation rate – highlighting why sophisticated return calculations matter for preserving and building actual wealth.

Types of Investment Return Calculations

Simple Return

Simple return, also known as the arithmetic return, is the most basic method for calculating investment performance.

Formula: Simple Return = (Ending Value – Beginning Value) / Beginning Value × 100%

For example, if you invest $10,000 and it grows to $12,000 in one year, the simple return is: ($12,000 – $10,000) / $10,000 × 100% = 20%

Advantages:

Easy to calculate and understand
Suitable for short-term investments with no cash flows
Provides a quick performance snapshot

Limitations:

Doesn’t account for the timing of cash flows
Can be misleading for long-term performance evaluation
Doesn’t consider compounding effects

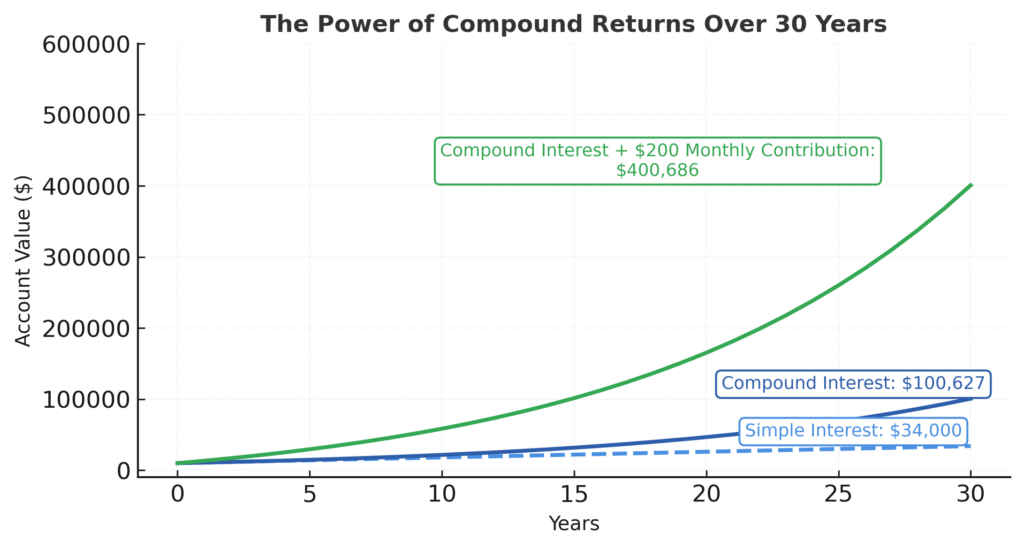
Compound Annual Growth Rate (CAGR)

CAGR provides a smoothed annualized return over a multi-year period, accounting for compounding effects.

Formula: CAGR = (Ending Value / Beginning Value)^(1/n) – 1

Where n is the number of years.

For example, if a $10,000 investment grows to $16,105 over 5 years, the CAGR is: ($16,105 / $10,000)^(1/5) – 1 = 10%

Advantages:

Accounts for compounding effects
Provides a standardized annual rate for multi-year investments
Enables fair comparison between investments with different time horizons

Limitations:

Assumes constant growth rate (smooths volatility)
Doesn’t account for interim cash flows
May mask significant performance variations within the period

Internal Rate of Return (IRR)

IRR is the discount rate that makes the net present value (NPV) of all cash flows equal to zero. It’s particularly useful for investments with irregular cash flows.

Formula: 0 = CF₀ + CF₁/(1+IRR)¹ + CF₂/(1+IRR)² + … + CFₙ/(1+IRR)ⁿ

Where CF represents cash flows (negative for investments, positive for returns).

Advantages:

Accounts for both timing and magnitude of all cash flows
Considers the time value of money
Ideal for complex investments like real estate, private equity, or venture capital

Limitations:

More complex to calculate (usually requires software)
Can produce multiple solutions for certain cash flow patterns
May overstate performance for investments with early positive cash flows

Money-Weighted Return (MWR)

Also known as the dollar-weighted return, MWR is equivalent to IRR and measures the performance of an investment considering the size and timing of cash flows.

Advantages:

Reflects the actual investor experience including timing decisions
Accounts for cash flow impacts on overall performance
Useful for assessing personal investment performance

Limitations:

Makes it challenging to compare investment manager performance
Influenced by the timing of deposits and withdrawals
May attribute poor timing decisions to investment performance

Time-Weighted Return (TWR)

TWR measures investment performance independent of external cash flows, making it ideal for evaluating investment manager performance.

Formula: TWR = [(1 + R₁) × (1 + R₂) × … × (1 + Rₙ)] – 1

Where R represents returns between valuation points.

Advantages:

Eliminates the impact of cash flow timing decisions
Industry standard for evaluating investment managers
Allows fair comparison between different managers

Limitations:

May not reflect an individual investor’s actual experience
Requires frequent portfolio valuations, especially around cash flow events
More complex to calculate than simple returns

Real Return vs. Nominal Return

Nominal Return represents the return without accounting for inflation.

Real Return adjusts for inflation to reflect the actual purchasing power gained.

Formula: Real Return ≈ Nominal Return – Inflation Rate

For example, if an investment generates a 9% nominal return during a period with 4% inflation: Real Return ≈ 9% – 4% = 5%

Significance:

Real returns provide a more accurate picture of wealth-building potential
Essential for long-term financial planning
Allows for meaningful historical comparisons across different inflation environments

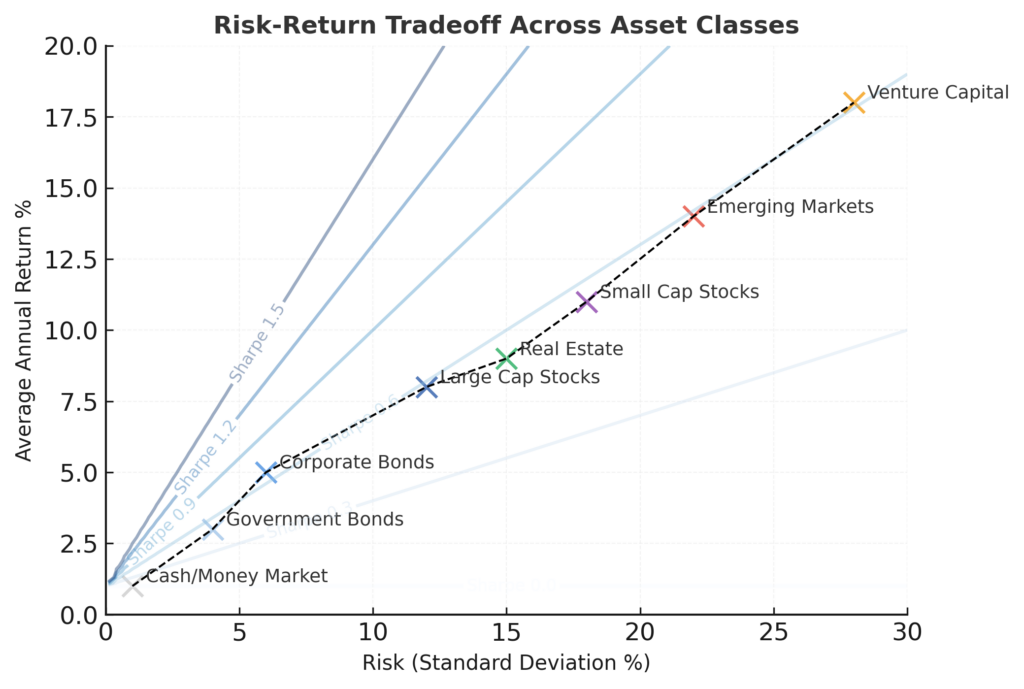
Risk-Adjusted Returns

These metrics evaluate investment performance relative to the risk taken.

Sharpe Ratio

Measures excess return per unit of risk.

Formula: Sharpe Ratio = (Investment Return – Risk-Free Rate) / Standard Deviation of Investment Returns

Sortino Ratio

Similar to Sharpe, but only penalizes downside risk.

Treynor Ratio

Measures excess return per unit of market risk (beta).

Importance:

Provides context for raw return numbers
Helps identify investments that deliver better returns for the level of risk
Essential for constructing optimized portfolios

Comparison of Return Calculation Methods

Return Metric	Best Used For	Advantages	Limitations	Typical Users
Simple Return	Short-term investments, Quick assessments	Easy to calculate, Intuitive	Ignores compounding, Timing of flows	Individual investors, Media reporting
CAGR	Multi-year investments without cash flows	Accounts for compounding, Standardizes periods	Smooths volatility, Ignores interim flows	Financial analysts, Fund marketing
IRR/MWR	Complex investments with irregular cash flows	Accounts for flow timing, Measures investor experience	Complex calculations, Multiple solutions possible	Real estate investors, Private equity
TWR	Evaluating investment manager performance	Eliminates impact of client deposit/withdrawal timing	May not match investor experience	Professional asset managers, Institutional investors
Risk-Adjusted (Sharpe, Sortino)	Portfolio optimization, Manager selection	Incorporates risk assessment, Better for comparisons	Requires statistical knowledge, Assumes normal distributions	Sophisticated investors, Institutional allocators

Benefits of Mastering Return Calculations

More Accurate Performance Evaluation

Understanding sophisticated return calculations enables investors to assess performance more accurately across different asset classes and time periods. This precision prevents both unwarranted complacency and unnecessary panic when reviewing investment results.

For example, an investor comparing two mutual funds might see that Fund A returned 45% over three years while Fund B returned 42%. Without calculating annualized returns, the investor might miss that Fund A’s returns were concentrated in year one followed by underperformance, while Fund B showed consistent growth – indicating potentially superior management at Fund B.

Better Investment Decision-Making

Armed with proper return metrics, investors can make more informed decisions about asset allocation, manager selection, and specific investment opportunities.

A wealth manager using risk-adjusted return metrics might determine that a hedge fund returning 12% annually with high volatility (Sharpe ratio of 0.7) is actually inferior to a diversified ETF portfolio returning 10% with lower volatility (Sharpe ratio of 1.2) – insight that would be missed by focusing solely on raw returns.

Enhanced Communication with Advisors

Investors who understand return calculations can engage more effectively with financial advisors, asking pertinent questions and critically evaluating recommendations.

Realistic Expectations and Goal Setting

By understanding historical returns in proper context, investors can set realistic expectations and create achievable financial goals based on sound mathematical principles rather than marketing claims.

According to a J.P. Morgan analysis, investors who based their retirement planning on simple average returns often underestimated required savings by 25-30% compared to those who used more sophisticated return models accounting for sequence risk and withdrawal patterns.

Tax Efficiency Optimization

Advanced return calculations that incorporate tax impacts allow investors to optimize after-tax returns, which ultimately determine actual wealth accumulation.

A high-income investor comparing municipal bonds yielding 4% (tax-free) with corporate bonds yielding 5.2% (taxable at 37%) would recognize the municipal bonds offer superior after-tax returns of 4% versus 3.28% – a distinction only visible when calculating tax-adjusted returns.

Challenges and Risks in Return Calculations

Data Accuracy and Availability

Calculating accurate returns requires comprehensive and precise data, which can be difficult to obtain, especially for alternative investments or complex portfolios spanning multiple accounts.

Misapplication of Methodologies

Using inappropriate return calculation methods for specific situations leads to misleading conclusions. This error often occurs when investors apply simple metrics to complex investment structures or vice versa.

Cognitive Biases

Humans have natural tendencies toward recency bias, confirmation bias, and innumeracy that can interfere with objective analysis of return data, regardless of calculation accuracy.

Market Volatility Distortions

Extreme market volatility can create distortions in short-term return calculations, potentially leading to flawed long-term decisions if not properly contextualized.

During the March 2020 COVID-19 market crash, many investments showed dramatic negative returns that, if annualized using simple methods, suggested catastrophic long-term projections that proved wildly inaccurate for investors who maintained positions through the recovery.

Benchmark Selection Problems

Choosing inappropriate benchmarks for comparison can lead to misleading conclusions about relative performance, even when return calculations themselves are accurate.

A small-cap value fund manager showing 12% annual returns might appear unsuccessful when compared to the S&P 500’s 15% return during a period when large-cap growth stocks dominated, despite outperforming their appropriate small-cap value benchmark by 3 percentage points.

Software and Calculator Limitations

Investment apps and calculators may use simplified return methodologies that don’t capture important nuances, especially for complex portfolios.

How to Calculate Returns: Step-by-Step Implementation

Simple Return Calculation

Determine your beginning investment value
Identify your ending investment value
Subtract beginning value from ending value
Divide the result by the beginning value
Multiply by 100 to convert to a percentage

Example:

Beginning value: $25,000
Ending value: $28,750
Return: ($28,750 – $25,000) / $25,000 × 100% = 15%

CAGR Calculation

Determine your beginning investment value
Identify your ending investment value
Determine the number of years
Divide ending value by beginning value
Raise the result to the power of (1/number of years)
Subtract 1 and multiply by 100 to get percentage

Example:

Beginning value: $50,000
Ending value after 4 years: $77,716
CAGR: ($77,716 / $50,000)^(1/4) – 1 = 11.67%

IRR Calculation

List all cash flows with dates (negative for investments, positive for returns)
Use financial calculator, spreadsheet function (e.g., XIRR in Excel), or specialized software
Verify the result makes logical sense

Example:

Initial investment (Year 0): -$100,000
Year 1 cash flow: $5,000
Year 2 cash flow: $8,000
Year 3 cash flow: $12,000
Year 4 cash flow: $15,000
Year 5 final value plus cash flow: $130,000
IRR: 10.37%

TWR Calculation

Value the portfolio immediately before and after each cash flow
Calculate the return between each valuation point
Link these returns by multiplying (1 + R) for each period
Subtract 1 from the result

Example:

Period 1 return: 5%
Period 2 return: -2%
Period 3 return: 8%
TWR: [(1 + 0.05) × (1 – 0.02) × (1 + 0.08)] – 1 = 11.05%

Risk-Adjusted Return Calculation (Sharpe Ratio)

Calculate the investment’s average return over period
Determine the risk-free rate for the same period
Calculate the standard deviation of investment returns
Subtract risk-free rate from investment return
Divide by standard deviation

Example:

Average annual return: 12%
Risk-free rate: 3%
Standard deviation: 15%
Sharpe Ratio: (12% – 3%) / 15% = 0.60

Tools and Resources for Return Calculations

Spreadsheet Applications

Microsoft Excel: XIRR, RATE, IRR functions
Google Sheets: Similar functionality to Excel with cloud-based accessibility

Financial Calculators

Texas Instruments BA II Plus: Industry standard for financial calculations
HP 12C: Popular among financial professionals

Investment Platforms

Morningstar: Provides comprehensive return metrics for mutual funds and ETFs
Portfolio Visualizer: Online tool for sophisticated portfolio analysis and return calculations

Professional Software

Bloomberg Terminal: Industry-leading financial analysis platform
FactSet: Comprehensive investment analysis tools
Refinitiv Eikon: Financial analysis and calculation tools

Mobile Applications

Personal Capital: Tracks investment performance across accounts
Portfolio Performance: Open-source portfolio tracking and return calculation

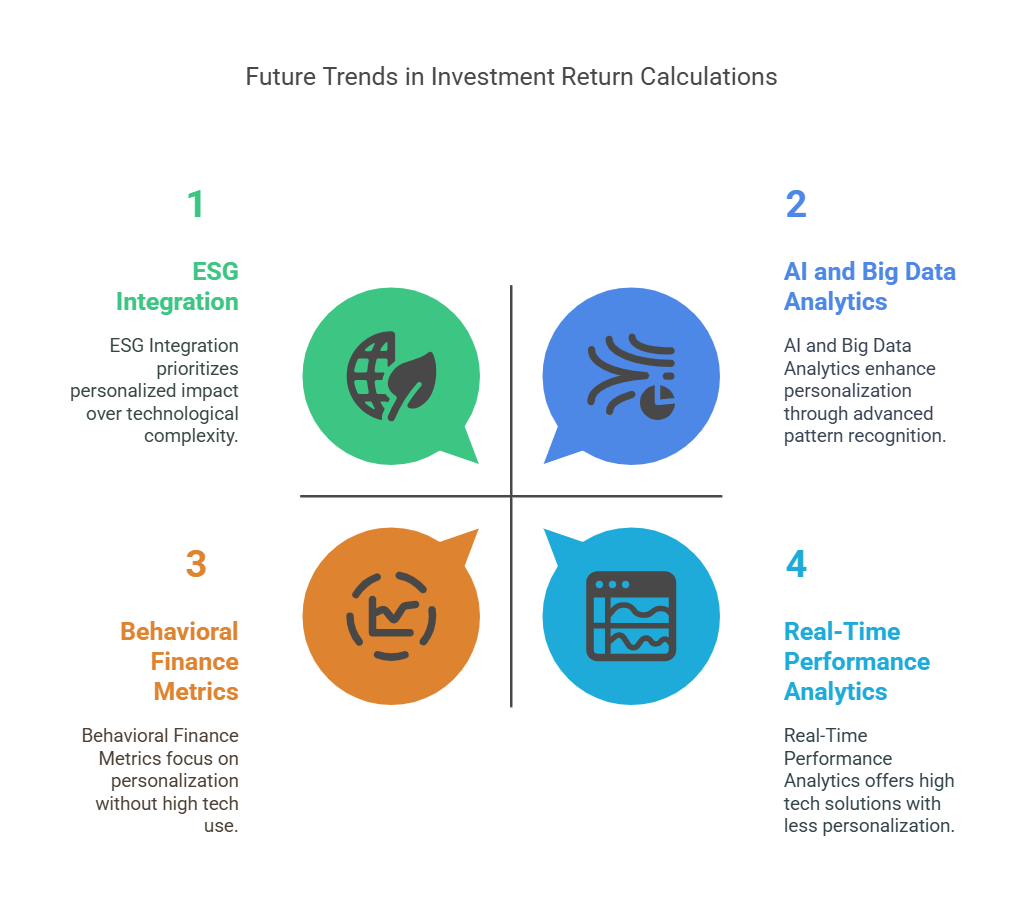
Future Trends in Investment Return Calculations

Increased Granularity and Customization

Return calculations are becoming increasingly personalized, with metrics tailored to specific investor goals, time horizons, and risk tolerances rather than standardized benchmarks. By 2026, expect to see mainstream investment platforms offering goal-based return tracking that dynamically adjusts based on individual financial objectives.

Integration of Environmental, Social, and Governance (ESG) Factors

Return calculations are evolving to incorporate ESG impact alongside financial performance. According to Morgan Stanley research, 78% of institutional investors now integrate ESG considerations into their return assessment frameworks – a trend rapidly spreading to retail investor tools.

Artificial Intelligence and Big Data Analytics

Machine learning algorithms are enhancing return calculations by identifying subtle patterns and correlations that traditional methods miss. JP Morgan’s AI-powered return analysis system detected that traditional metrics underestimated the impact of sentiment shifts on emerging market returns by approximately 2.3 percentage points annually.

Real-Time Performance Analytics

Technological advances are enabling continuous real-time return calculations rather than periodic snapshots, giving investors more timely information for decision-making. Leading wealth management platforms now offer intraday performance metrics that incorporate multiple return calculation methodologies simultaneously.

Blockchain-Based Verification

Blockchain technology is beginning to revolutionize return verification by creating immutable records of transaction history and performance claims. This transparency will become increasingly important as investors demand greater accountability from investment managers and platforms.

Integration of Behavioral Finance Metrics

Return calculations are starting to incorporate behavioral factors, recognizing that investor behavior significantly impacts actual realized returns. Vanguard’s Advisor’s Alpha research shows that behavioral coaching alone can add approximately 1.5% in net returns annually by helping investors avoid common timing mistakes.

FAQs – Investment Return Calculation

1. What is the difference between arithmetic average return and geometric average return?

Arithmetic average return is the simple mean of periodic returns, calculated by summing all returns and dividing by the number of periods. Geometric average return (CAGR) accounts for compounding by linking returns multiplicatively. For volatile investments, arithmetic averages will always be higher than geometric averages. For example, an investment that gains 50% in year one and loses 40% in year two has an arithmetic average of +5% annually but a geometric average of -7.3% annually.

2. Why might two investors in the same fund experience different returns?

Two investors can experience different returns in the same fund due to the timing of their cash flows. If Investor A invested a lump sum before a market upswing while Investor B dollar-cost averaged through both up and down periods, their money-weighted returns would differ significantly despite holding the same fund. This is why personal investment returns often diverge from published fund returns.

3. How do dividends and distributions affect return calculations?

Dividends and distributions increase total return when reinvested but must be properly accounted for in calculations. Total return includes both price appreciation and income (dividends/interest), while price return excludes income. For example, a stock that appreciates 7% while paying a 3% dividend yield actually provides a 10% total return if dividends are reinvested – a significant difference that compounds dramatically over time.

4. What return metric should I use when comparing different types of investments?

When comparing different investment types, use risk-adjusted returns like the Sharpe ratio for the most meaningful comparison, as raw returns without context can be misleading. For investments with similar risk profiles but different cash flow patterns, IRR provides the most accurate comparison by accounting for the timing of cash flows.

5. How do fees impact investment returns?

Fees directly reduce investment returns and compound negatively over time. A seemingly small difference between a 0.1% and 1% expense ratio on an index fund represents approximately 24% less wealth over 30 years on a $100,000 investment growing at 7% annually. Always calculate returns net of all fees for accurate performance assessment.

6. Can past returns predict future performance?

Past returns alone have limited predictive value for future performance. Studies consistently show little persistence in outperformance beyond what would be expected by chance. However, risk-adjusted metrics like Sharpe ratio show somewhat better persistence. Focus on understanding the drivers behind returns rather than extrapolating past performance.

7. How should I calculate returns for a portfolio with multiple asset classes?

For a multi-asset portfolio, calculate time-weighted returns for the entire portfolio to evaluate overall performance. Additionally, calculate the returns for each asset class separately to assess their individual contributions. Attribution analysis can then determine whether performance came from asset allocation decisions or security selection within asset classes.

8. What is a good annual return on investments?

“Good” returns vary based on risk level, time period, and comparison to appropriate benchmarks. Historically, U.S. large-cap stocks have returned approximately 10% annually before inflation (7% real returns), while bonds have returned about 5% nominally (2-3% real). Higher-risk investments should deliver higher returns to compensate for additional volatility.

9. How do taxes affect investment return calculations?

Taxes can significantly reduce effective returns, especially for high-income investors. When comparing taxable and tax-advantaged investments, calculate after-tax returns by applying your marginal tax rate to interest, dividends, and realized capital gains. For example, an investor in the 35% tax bracket would keep only $65 of every $100 in taxable interest, effectively reducing a 5% yield to 3.25%.

10. How should I account for inflation in my return calculations?

To account for inflation, subtract the inflation rate from nominal returns to determine real returns. For long-term planning, using real returns provides a more accurate picture of future purchasing power. For example, the historical 10% nominal return on U.S. stocks translates to approximately 7% after accounting for the long-term inflation average of 3%.

Conclusion

Mastering investment return calculations is an essential skill for serious wealth builders in today’s complex financial landscape. By understanding and appropriately applying different return metrics – from simple returns to sophisticated measures like time-weighted and risk-adjusted returns – investors gain valuable insights that drive better decision-making and ultimately lead to superior outcomes.

The future of return calculations is increasingly sophisticated, with artificial intelligence, real-time analytics, and customized metrics tailored to individual investor goals. However, the fundamental principles remain constant: accurate return assessment requires proper methodology selection, consistent application, and thoughtful interpretation within the context of risk, time horizon, and personal financial objectives.

By applying the formulas and approaches outlined in this guide, investors can move beyond simplistic performance snapshots to develop a nuanced understanding of their investments’ true effectiveness. This knowledge empowers more informed asset allocation, manager selection, and portfolio optimization – the building blocks of sustainable wealth creation in an increasingly complex financial world.

For your reference, recently published articles include:

………………………………………………..

Important Notice: The information in this article is for general and public information purposes only. It solely reflects Didi Somm’s or his Staff’s opinion, and no responsibility can be assumed for errors or omissions in the service’s contents. For details, please check the Disclaimer at the bottom of the homepage.